To convert a number from complex form to polar form you have simply to apply the definitions of sin and cos.
First of all we use the real part of the number (#3sqrt(3)#) as the #x# coordinates and the imaginary part (without the #i#, so only #3#) as the #y# and we put this on two axis as in the figure.
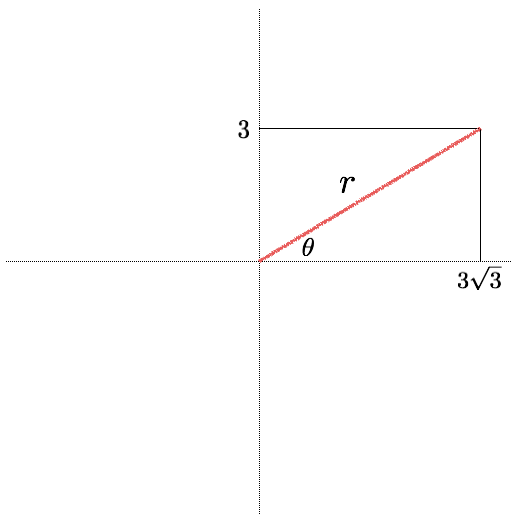
The polar form is nothing but #r# and #theta#.
#r# can be obtained easily applying the Pitagora's theorem:
#r=sqrt((3sqrt(3))^2+3^2)=sqrt(27+9)=sqrt(36)=6#.
#theta# can be calculated using the definition of cosine or sine.
We know that #x=rcos(theta)# and #y=rsin(theta)#. We could then say, for example, that #sin(theta)=y/r# and then #theta=arcsin(y/r)#.
In the same way we can say #theta=arccos(x/r)#. But my favorite consists in dividing the two coordinates having
#y/x=sin(theta)/cos(theta)# and because #sin(theta)/cos(theta)=tan(theta)# we have #y/x=tan(theta)# and finally
#theta=arctan(y/x)#. In our case #theta=arctan(3/{3sqrt(3)})=arctan(1/sqrt(3))\approx0.52#.
Then your polar coordinates are #(6, 0.52)#.
To see if the result is correct, you can transform back your number as:
#rcos(theta)+irsin(theta) = 6cos(0.52)+i*6sin(0.52)=5.19+3i# and 5.19 is the approximation of #3sqrt(3)#.


