The length of a rectangle is 4 inches more than its width, and its perimeter is 34 inches. What is the length and width of the rectangle?
2 Answers
Length
Explanation:
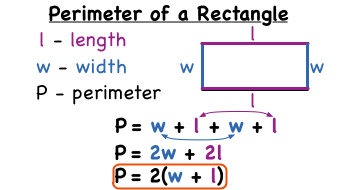
Perimeter
Given
length is
width is
Explanation:
Let length be
Let width be
Let perimeter be
First, we must construct an equation for these variables:
But, Perimeter of a rectangle
So:
But, since
Solve for
Now, we can substitute
becomes:
Solve for
Thus, length is
Thus, width is

