Question

How do you solve `3(x-7) = 6(x-10)`?

Answer 1

`x = 13`

Explanation:

`3(x-7) = 6(x-10)`

Use the distributive property (shown below) to simplify each side:

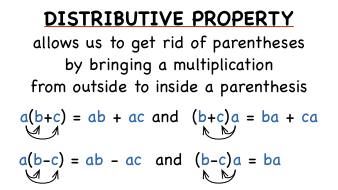
Following this image, we know that:
`color(blue)(3(x-7) = (3 * x) + (3 * -7) = 3x - 21)`
and
`color(blue)(6(x-10) = (6 * x) + (6 * -10) = 6x - 60)`

Put them back into the equation:
`3x - 21 = 6x - 60`

Subtract `color(blue)6x` from both sides:
`3x - 21 quadcolor(blue)(-quad6x) = 6x - 60 quadcolor(blue)(-quad6x)`

`-3x - 21 = -60`

Add `color(blue)21` on both sides:
`-3x - 21 quadcolor(blue)(+quad21) = -60 quadcolor(blue)(+quad21)`

`-3x = -39`

Divide both sides by `color(blue)(-3)`:
`(-3x)/color(blue)(-3) = (-39)/color(blue)(-3)`

Therefore,
`x = 13`

Hope this helps!

Answer 2

`x=13`

Explanation:

We can divide both sides by `3` to get

`x-7=2(x-10)`

Next, we can distribute the `2` on the right to get

`x-7=2x-20`

Next, we can add `7` to both sides to get

`x=2x-13`

To get our constants on one side, we can subtract `2x` from both sides to get

`-x=-13`

Lastly, we can divide both sides by `-1` to get

`x=13`

Hope this helps!