Question #755fb
2 Answers
The surface area of a hemisphere having a volume of
Explanation:
From https://www.khanacademy.org/math/geometry/hs-geo-solids/hs-geo-solids-intro/v/volume-of-a-sphere the Volume of a Sphere is:
Therefore the volume of a hemisphere is 1/2 of this or:
So to determine
https://www.math.hmc.edu/funfacts/ffiles/20004.2-3.shtml the surface area of a sphere is:
Therefore the surface are of a hemisphere is 1/2 of this or:
We know the radius of the square is
I got
Explanation:
The area of a sphere is
The volume of the entire sphere is
where the volume of the hemisphere is given as
We can use this value into the expression for half the surface of the sphere BUT....do we need also to consider the base of the hemisphere?
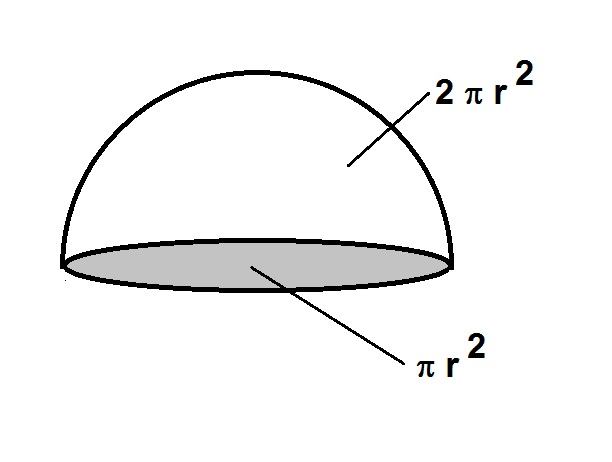
Considering the base as well we get:


