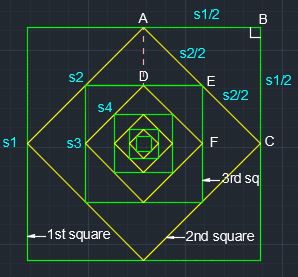
Hopefully, my interpretation of the question is correct. If it is wrong, do let me know. Thank you.
Let #s_1,s_2,s_3,s_4,s_5,s_6,s_7,s_8,s_9# be the side length of the 1st square, 2nd square, 3rd square, ..... 8th square, and 9th square, respectively.
Given #s_1=6# units.
As can be seen in the diagram,
In #DeltaABC#, as #AB=BC=6/2=3, and angleABC=90^@#
#=> AC=3sqrt2, => s_2=3sqrt2#
#=> AE=EC=(3sqrt2)/2#
In #DeltaADE#, as #AE=(3sqrt2)/2, and angleDAE=angleDEA=45^@#,
#=> AD=DE=3/2, => s_3=2*DE=2xx3/2=3#
Note that :
#s_1=6, s_1/2=3#,
#s_2=(s1)/2sqrt2=3sqrt2#,
#s_3=(s_2)/sqrt2=(3sqrt2)/sqrt2=3=s_1/2#
As this pattern repeats :
#=> s_5=s_3/2=3/2#
#=> s_7=s_5/2=3/4#
#=> s_9=s_7/2=3/8#
Hence, perimeter of the ninth square #=4*s_9=4*3/8=3/2=1.5# units
Footnote : #DeltaABC, DeltaADE, DeltaDEF# .... are all isosceles right triangles, with angles of #45^@, 45^@, and 90^@#, and sides in the ratio of #1 : 1: sqrt2#.
