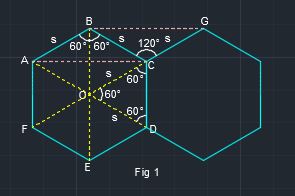
As shown in Fig 1, #ABCDEF# is a regular hexagon.
A regular hexagon can be divided into 6 equilateral triangles.
Let #A_e# be the area of each equilateral triangle and #A_h# be the area of the hexagon.
#=> A_h=6A_e#
#OABC# is a rhombus consisting of two equilateral triangles,
Area #OABC=2A_e#,
Diagonal #AC# divides the rhombus into two congruent isosceles triangles, #DeltaABC, and DeltaAOC#
#=># Area #DeltaABC=A_e#
Area #DeltaBCG=# Area#ABC=A_e=1/6A_h#

As shown in Fig 2,
the rectangle mainly consists of hexagons placed side by side.
Let #a# be the area of one regular hexagon in the rectangle.
#=># Total area #A_t= (10+4*1/2+8*1/6)a=(40a)/3#
Given that the area of the rectangle is #80# sq. ft
#=> A_t=80#
#=> (40a)/3=80#
#=># area of one hexagon #a=(80*3)/40=6# sq. ft.
