In a regular polygon, apothem is the length of the line segment from the center of the regular polygon to the midpoint of a side.
As we have a polygon with #18# sides, each side subtends an angle of #20^@# at the center forming an isosceles triangle and area of polygon will be #18# times the area of this polygon. Now consider the following figure.
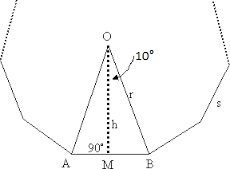
Let #h# be the apothem, then #MB=hxxtan10^@# and #AB=2hxxtan10^@# and area of isosceles triangle is
#(2hxxtan10^@xxh)/2=h^2tan10^@# and area of polygon is
#18h^2tan10^@# and as #h=15.5#
Area of polygon is #18xx15.5^2xxtan10^@#
= #18xx15.5^2xx0.176327=762.526#
and perimeter is #18AB=18xx15.5xx0.176327=49.195#
Note - we can say that if #a# is the length of the apothem of a polygon of #n# sides, its perimeter is #2natan(pi/n)# and area is #na^2tan(pi/n)#.

