Question

A cone has a height of `12 cm` and its base has a radius of `7 cm`. If the cone is horizontally cut into two segments `3 cm` from the base, what would the surface area of the bottom segment be?

Answer 1

`:.color(purple)(=374.19cm^2` to the nearest `cm^2`

Explanation:

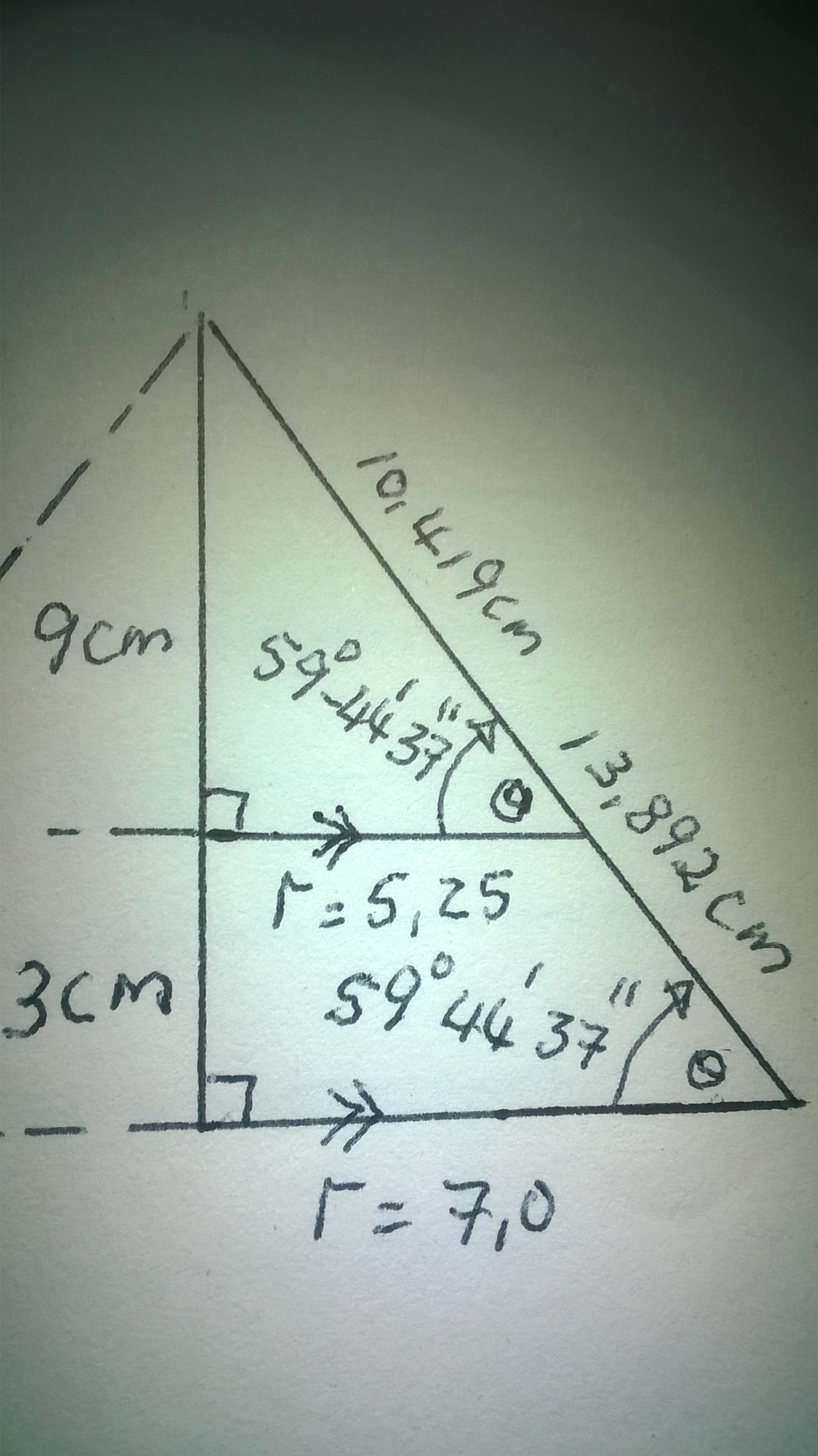
:.Pythagoras: `c^2=12^2+7^2`

`:.c=L=sqrt(12^2+7^2)`

`:. c=Lcolor(purple)(=13.892cm`

`:.12/7=tan theta=1.714285714=59^@44’37”`

:.`color(purple)(S.A.`=pirL#

:.S.A.`=pi*7*13.892`

:.S.A.`=305.501`

:.Total S.A.`color(purple)(=305.501cm^2`

`:.Cot 59^@44’37”*9=5.25cm=`radius of top part

:.Pythagoras: `c^2=9^2+5.25^2`

`:.c=L=sqrt(9^2+5.25^2)`

`:. c=Lcolor(purple)(=10.419cm` top part

:.S.A. top part`=pi*r*L`

S.A. top part`:.pi*5.25*10.419`

S.A. top part`:.=171.844`

S.A. top part`:.color(purple)(=171.844cm^2`

:.S.A. Bottom part`color(purple)(=305.501-171.844=133.657cm^2`

:.S.A. Bottom part`=133.657+pi7^2+pi5.25^2=374.1851875cm^2`

`:.color(purple)(=374.19cm^2` to the nearest 2 decimal places `cm^2`

Answer 2

`TSA = 374.19cm^2`

Explanation:

We can use similar figures to answer parts of this question.

It is the same cone, so the angles are all equal and the sides are in the same ratio.
We can therefore compare the heights and the radii.

The Height of the larger cone `H= 12cm`

The height of the smaller cone: `h = 12-3 = 9cm`

The Curved Surface Area of the larger cone:

`A = piRL`

`L` is the slant height and is found from Pythagoras' Theorem:

`L = sqrt(7^2 + 12^2) = sqrt193`

`A = 7pisqrt193`

Use similar figures to find the radius of the smaller cone with a height of `9`.

`r/7 = 9/12`

`r = (9xx7)/12 = 5.25`

Find the slant height:

`l= sqrt(5.25^2 +9^2) = sqrt(108.5625)`

The curved area is `a = pirl`

`a = 5.25pisqrt108.5625`

The curved surface area of the bottom part (fustrum) is the difference between the curved surfaces calculated above,

`A = 7pisqrt193-5.25pisqrt108.5625`

`A= 133.66cm^2`

The total surface area is made up of the curved surface area plus the areas of the upper and lower circles.

`TSA = 133.66 + pi7^2 +pi5.25^2`

`TSA = 374.19cm^2`