Question

A cone has a height of `18 cm` and its base has a radius of `6 cm`. If the cone is horizontally cut into two segments `3 cm` from the base, what would the surface area of the bottom segment be?

Answer 1

200.9cm^2

Explanation:

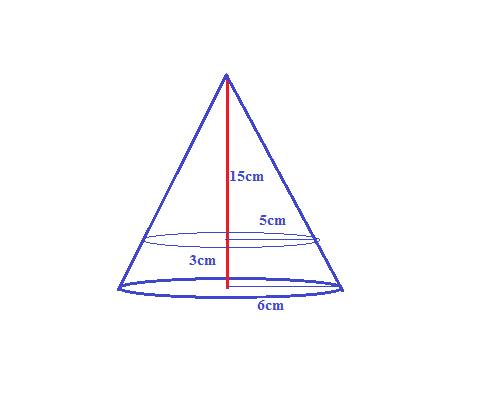
For Original larger cone

The radius of the cone =`R=6cm`

The height of the cone =`H=18cm`

The slant height of the cone

=`L=sqrt(H^2+R^2)=sqrt(18^2+6^2)=6sqrt10cm`

The surface area of larger cone

`A_b=pixxRxxL=pixx6xx6sqrt10=36sqrt10picm^2`

~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
For the smaller cone (cut off)

The height of the smaller cone =`h=(18-3)cm=15cm`

If the radius of the smaller cone be r ,then

`r/h=R/H=>r=h/HxxR=15/18xx6=5cm`

The slant height of the smaller cone

`=l=sqrt(h^2+r^2)=sqrt(15^2+5^2)=5sqrt10cm`

The Curved surface area of smaller cone

`A_s=pixxrxxl=pixx5xx5sqrt10=25sqrt10picm^2`

~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~

The Curved surface area of the bottom segment

`A_b-A_s=(36sqrt10pi-25sqrt10pi)=11sqrt10picm^2=109.3cm^2`

The total area of two circular planes of the bottom portion is
`A_c=pi(r^2+R^2)=pixx(5^2+6^2)=191.6cm^2`

The Total surface area of the bottom segment
`A_"Total"=(109.3+191.6)cm^2=200.9cm^2`