A cone has a height of #24 cm# and its base has a radius of #6 cm#. If the cone is horizontally cut into two segments #9 cm# from the base, what would the surface area of the bottom segment be?
1 Answer
Dec 2, 2017
Explanation:
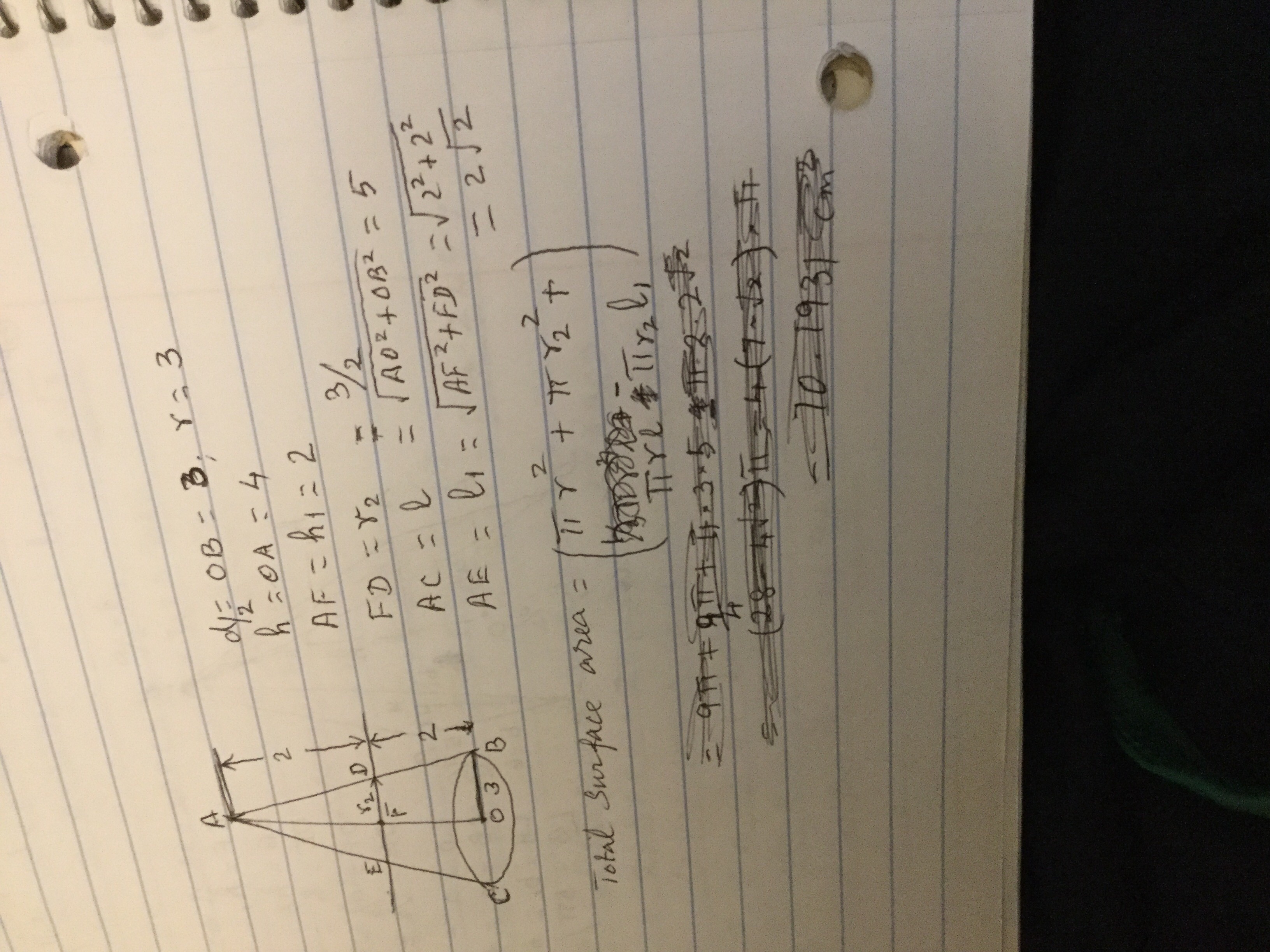
#pirl= pi* 6 * 24.7386 = 466.3116 cm^2
#pir_2l_1 = pi* 2.25 * 9.277 = 65.5752 cm^2
Total surface area =
