A cone has a height of #24 cm# and its base has a radius of #9 cm#. If the cone is horizontally cut into two segments #16 cm# from the base, what would the surface area of the bottom segment be?
1 Answer
Total Surface Area of the truncated cone
Explanation:
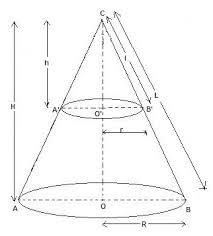
Given :
Lateral Surface Area of a cone
L S A of uncut cone
Similarly, L S A of cut cone is
L S A of truncated cone
Area of base of uncut cone
Area of base of cut cone
Total Surface Area of the truncated cone
