A pyramid has a base in the shape of a rhombus and a peak directly above the base's center. The pyramid's height is #2 #, its base has sides of length #1 #, and its base has a corner with an angle of #(2 pi)/3 #. What is the pyramid's surface area?

Question

1529 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-01-27T06:31:54

Total Surface Area #color(brown)(T S A) color(brown)(= 4.9892)#

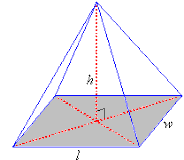
enter image source here
Pyramid's Total Surface Area (T S A) #A_T# =Lateral Surface Area + Rhombus Base Area #(A_R)#

Lateral Surface Area (L S A)= 4 * Area of Slant Triangle (A_S)

i.e. #A_T = A_B + (4 * A_S)#

To find #A_B, A_S#

Let a be the rhombus side, theta the corner angle and h the height of the pyramid.

Given : #a = 1, theta = (2pi)/3, h = 2#

#color(red)(A_R) = a^2 sin theta = 1^2 sin ((2pi)/3) color(red)(= sqrt3 /2 = 0.866)#

#A_S = (1/2) a * sqrt(h^2 + (a/2)^2)#

#color(red)(A_S) = (1/2)(1) * sqrt(2^2 + (1/2)^2) color(red)(= 1.0308)#

#color(red)(T S A) = A_T = A_R + (4 * A_S) = 0.866 + (4 * 1.0308) color(red)(= 4.9892)#