A pyramid has a parallelogram shaped base and a peak directly above its center. Its base's sides have lengths of #4 # and #1 # and the pyramid's height is #7 #. If one of the base's corners has an angle of #(5pi)/6#, what is the pyramid's surface area?

Question

1360 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-02-04T03:00:13

#T S A# #A_T = color(green)(37.1424)#

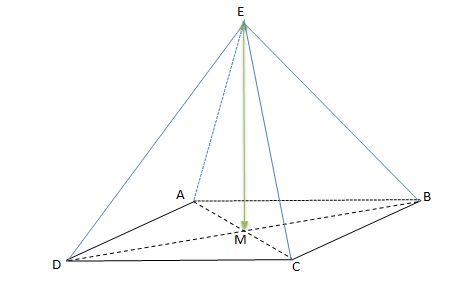
enter image source here

Total Surface Area of Pyramid #A_T# = Area of parallelogram base ABCD + 2 * ( Area of Triangles (AED + CED))

Let Area of ABCD as #A_p#, Area of AED as #A_(t1)#, Area of CED as #A_(t2)#

#A_p = a * b sin theta = 4 * 1 * sin ((5pi)/6) = 2#

#A_(t1) = (1/2) * 1 * l_1 = (1/2) * 1 * sqrt((a/2)^2 + h^2)#

#A_(l1) = (1/2) * 1 * sqrt(2/2)^2 + 7^2) = 3.5355#

Similarly, #A_(l2) = (1/2) * 4 * sqrt((1/2)*2 + 7^2) = 14.0357#

#T S A# #A_T= A_p + 2 * (A-(l1) + A_(l2)) = 2 + 2 * (3.5355 + 14.0357)#

#A_T = color(green)(37.1424)#