Question

A regular hexagon has side 2 meters and has a circumscribed circle. What is the area of the hexagon, and what is the area of the circumscribed circle?

Answer 1

The area of hexagon `=color(blue)(10.932 m^2`

area of the circle : `color(blue)(=12.56 m^2`

Explanation:

![)

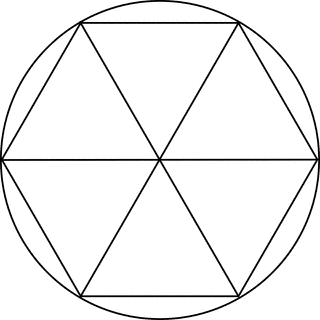
1) Hexagon
The formula for area of a hexagon is :
`color(blue)(area =(3sqrt3)/2 xx (side)^2`

The side `=2m`

`area =(3sqrt3)/2 xx (2)^2`

`=(3sqrt3)/cancel2 xxcancel4`

`=3sqrt3 xx2`

`=6sqrt3`
(`sqrt3 = 1.732)`

`=6xx1.732`

The area of hexagon `=color(blue)(10.932 m^2`

2) Circle

Observe the above diagram , each of the triangles within the hexagon are equilateral.

The angle around a point `=360^o`

So, the angle of each triangle `=360/6=60^o`

Now observe a triangle , `2` sides are radii of the circle itself and as per property : angles opposite equal sides are equal, so all angles of the triangle are`=60^o`.
Thus , the triangles are all equilateral .

The side of the hexagon `=` radii of the circle.

`r= 2m`

So, the area `=pi xx (r)^2`

`=3.14 xx 2^2`

`=3.14 xx 4`
area of the circle : `color(blue)(=12.56 m^2`