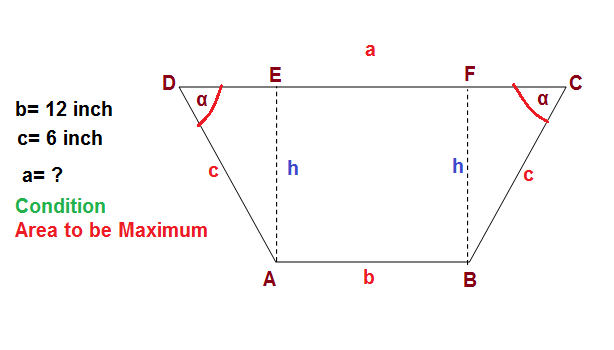
Let ABCD be an isosceles trapezoid having
one base #AB=b=12"inch"#
and legs #AD=BC=c=6"inch"#
other top base #CD=a=?#
and base angles #angle ADC=angle BCD=alpha#
Its height #AE=h#
Now from figure
#h=csinalpha and DE=CF=c cosalpha#
The area of the trapezium
#A=1/2(AB+CD)xxAE#
#=>A=1/2(AB+AB+2DE)xxADsinalpha#
#=>A=1/2(12+12+2xxADcosalpha)xxADsinalpha#
#=>A=1/2(12+12+2xx6cosalpha)xx6sinalpha#
#=>A=1/2(24+12cosalpha)xx6sinalpha#
#=>A=(12+6cosalpha)xx6sinalpha#
#=>A=36(2+cosalpha)sinalpha#
#=>A=36(2sinalpha+cosalphasinalpha)#
Differentiating w r to #alpha# we get
#(dA)/(dalpha)=36(2cosalpha+cos^2alpha-sin^2alpha)#
Imposing the condition of maximization of the are #A# i.e.#(dA)/(dalpha)=0# we get
#2cosalpha+cos^2alpha-sin^2alpha=0#
#=>2cosalpha+cos^2alpha-1+cos^2alpha=0#
#=>2cos^2alpha+2cosalpha-1=0#
So #cos alpha=(-2+sqrt(2^2-4xx2(-1)))/(2xx2)#
#cos alpha <-1# not possible , so other root neglected.
#=>cos alpha=(-2+sqrt12)/(2xx2)#
#=>cos alpha=(-2+2sqrt3)/(2xx2)#
#=>cos alpha=(sqrt3-1)/2#
So #a=CD=(AB+2DE)#
#=>a=(b+2xxcxxcosalpha)#
#=>a=(12+2xx6xx(sqrt3-1)/2)#
#=>a=(12+6xxsqrt3-6))#
#=>a=6(sqrt3+1)~~16.4# inch


