Question

Experimental/observational studies & relative frequencies?

Is the study experimental or observational?

9) "A clinic gives a drug to a group of ten patients and a placebo to another group of ten patients to find out if the drug has an effect on the patients' illness."
10) "A marketing firm does a survey to find out how many people use a product. Of the one hundred people contacted, fifteen said they use the product."

---

Provide an appropriate response. Round relative frequencies to thousandths.

11) "The preschool children at Elmwood Elementary School were asked to name their favorite color. The results are listed below. Construct a frequency distribution and a relative frequency distribution."

yellow, yellow, blue, purple, red, red, red, yellow, red, blue, red, blue, purple, purple, purple, blue, red, purple, red, green

Answer 1

See explanation.

Explanation:

An experimental study is one where a test of some sort needs to be given in order to produce a measurement. Neither the subject nor the experimenter could possibly know the exact outcome before it is measured. The outcome for each subject may also change if the test is done again. Good examples are when the effects of a pill are tested, or a speed test is given.

Observational studies are those where we simply observe a trait, opinion, or measurement for a subject; the subject may know the outcome before the observation is made, and the outcome for each subject is not expected to change through repeated observations. Good examples would be a questionnaire, opinion poll, or height/weight measurements.

9) is an example of an experimental study, while 10) is an example of an observational study.

A frequency distribution counts how many measurements fall within each of the categories the experimenter is interested in. It can be written as a table, but is most often expressed as a bar graph.

For 11), the frequency distribution could be written in a table, like this:

`7` Red
`3` Yellow
`1` Green
`4` Blue
`5` Purple

But since we tend to infer more useful information from a graph than a table, the bar graph for this data would look like this:

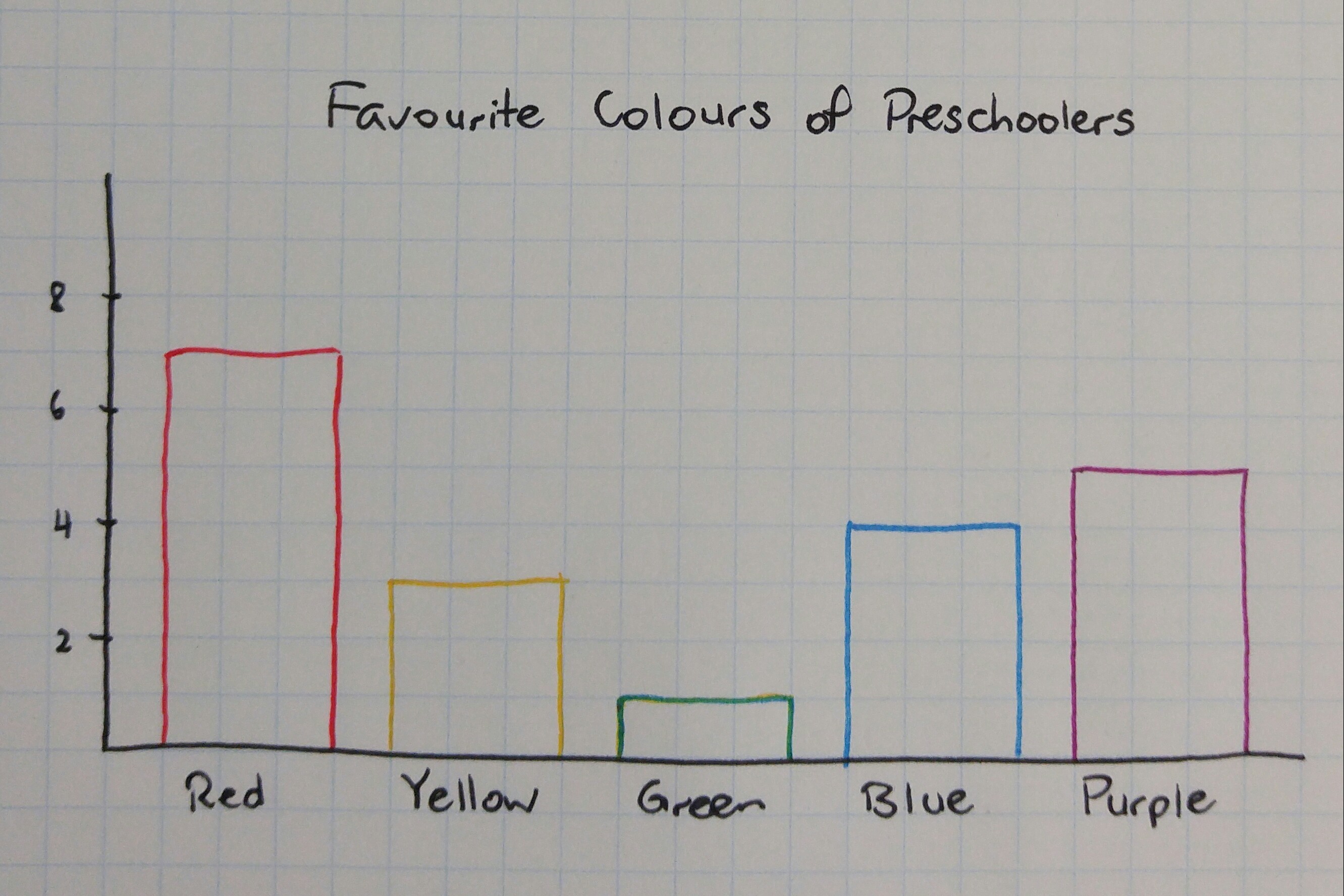
The relative frequencies are the ratios of the number in each category to the total number of measurements. For example, since there are 7 preschoolers whose favourite colour is red, and the total number of preschoolers surveyed is 20 (7+3+1+4+5), the relative frequency for red is `7/20`, or 0.350.

All of the relative frequencies are:

`0.350` Red
`0.150` Yellow
`0.050` Green
`0.200` Blue
`0.250` Purple

Notice how the sum of these numbers is `1`. This must be the case for all relative frequency tables, because we're listing the percentages of the class that named each colour as their favourite.