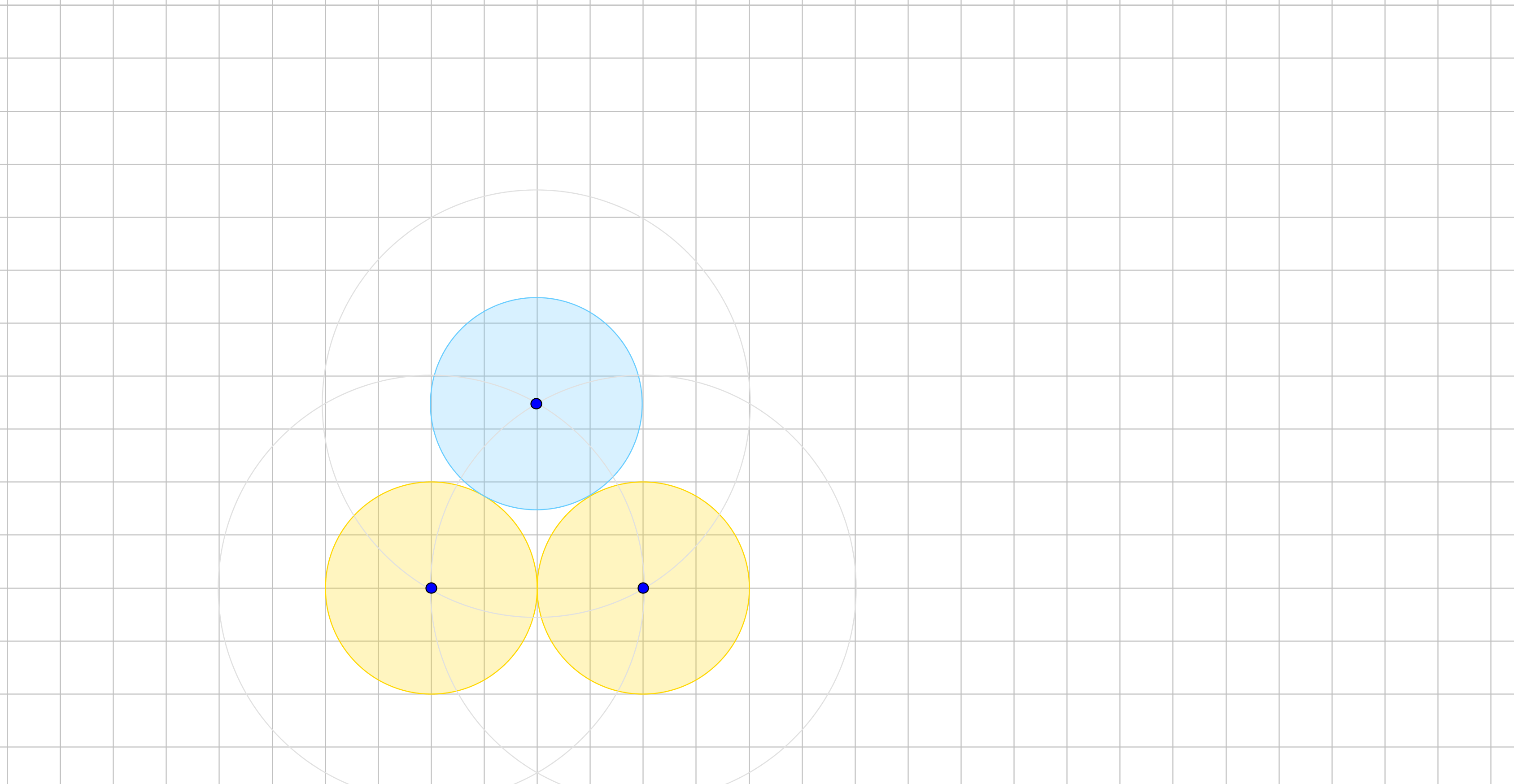
Given 3 circles with radius #r# positioned find the the radius #R# of the circumscribing circle in terms of the radius of the small circles #r#? If r = 5 cm what is the area of the circumscribing circle?

Question

3381 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-11-08T13:45:23

Radius of each of three circles touching each other is r.

The side of the equilateral triangle formed by joining the three centers of the given three circles is #=2r#

Height of this equlateral triangle is # =sqrt((2r)^2-r^2)=sqrt3r#

Radius of the circumcircle of this equilateral triangle is #=2/3xxsqrt3r=(2sqrt3r)/3#

Hence Radius of the circle cicumscribing three circles will be #R= (2sqrt3r)/3+r=((2sqrt3)/3+1)r#

Area of this circle

#=piR^2=pi( (2sqrt3)/3+1)^2r^2#

#=(4/3+1+(4sqrt3)/3)pir^2#

#=1/3(7+4sqrt3)xx3.14xx5^2cm^2#

#=364.45cm^2#