Question

How do you graph `y=1/2sqrtx`?

Answer 1

`" "`
Please read the explanation.

Explanation:

`" "`

Create adata table to generate the graph:

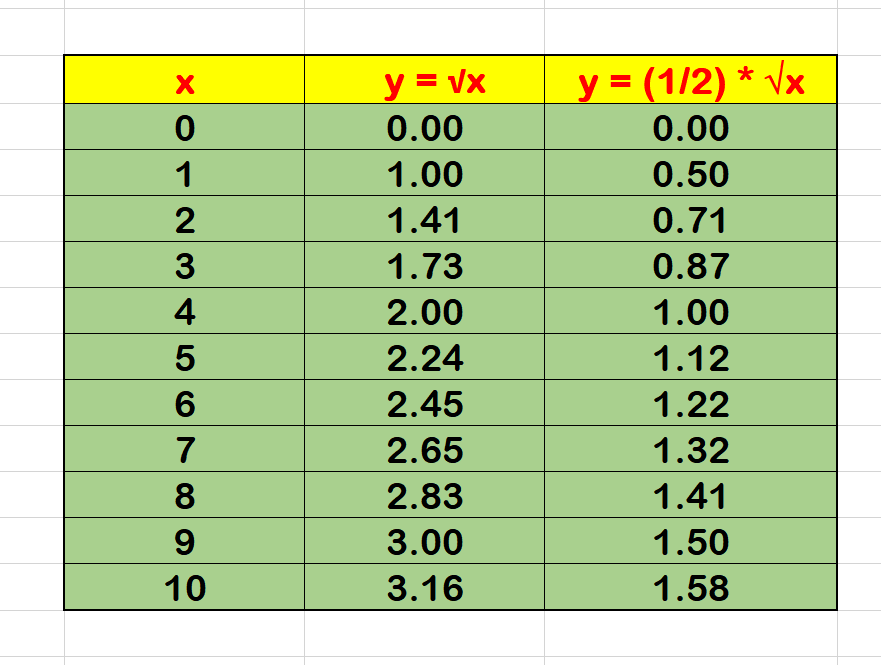
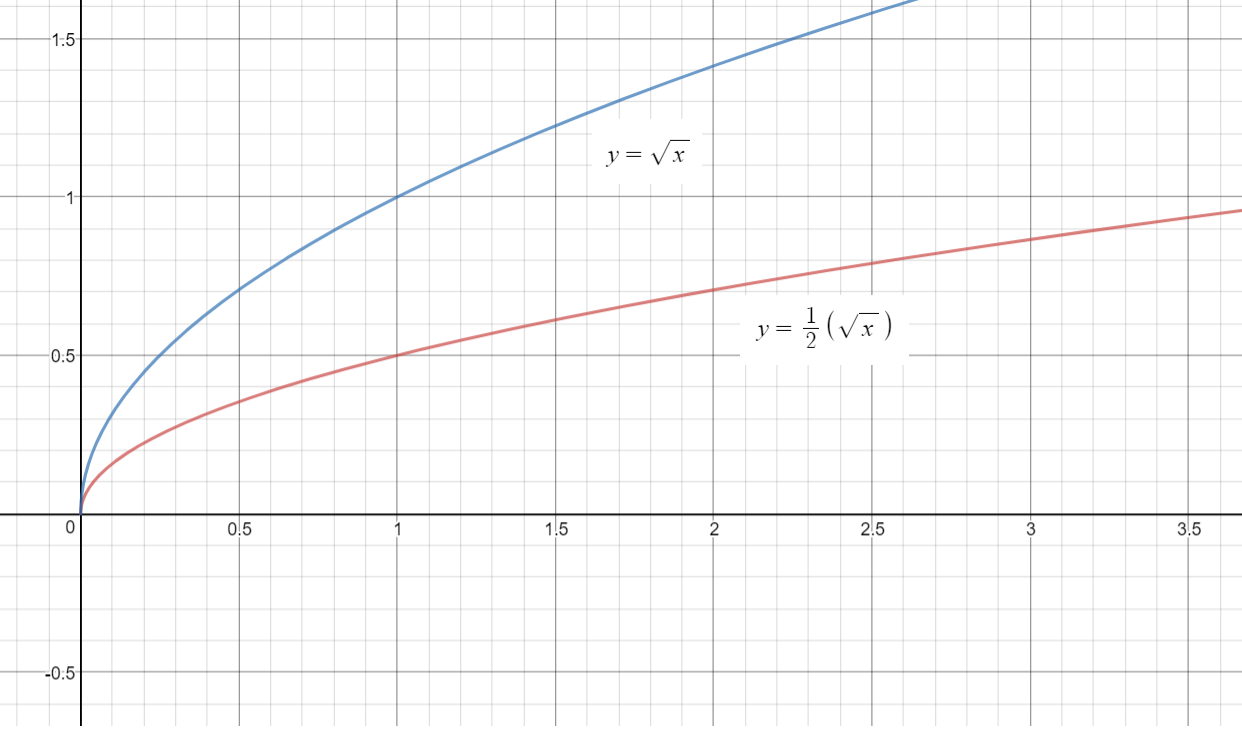
Observe the graphs given below:

1. Graph of `color(blue)(y=sqrt(x)`

2. Graph of `color(red)(y=(1/2)*sqrt(x)`

The radical function: `color(blue)(y=sqrt(x)` is the Parent Function:

The graph of the parent function starts at the origin `(0,0)`

The graph increases gradually.

The general form of the radical function:

`color(red)(y= f(x)=a sqrt[b(x-c)] +d`, where

( i). `color(red)(|a|` will stretch or shrink the graph vertically

( ii), `color(red)(|b|` will stretch or shrink the graph horizontally

(iii). `color(red)(c` will shift the graph left or right.

( iv). `color(red)(d` will shift the graph up or down.

( v). `color(red)(-a` will flip the graph across -axis

( vi). `color(red)(-b` will flip the graph across y-axis

We can compare both the graphs (parent function and the function given) to comprehend the behavior of the graph and the corresponding radical function.

Hope it helps.