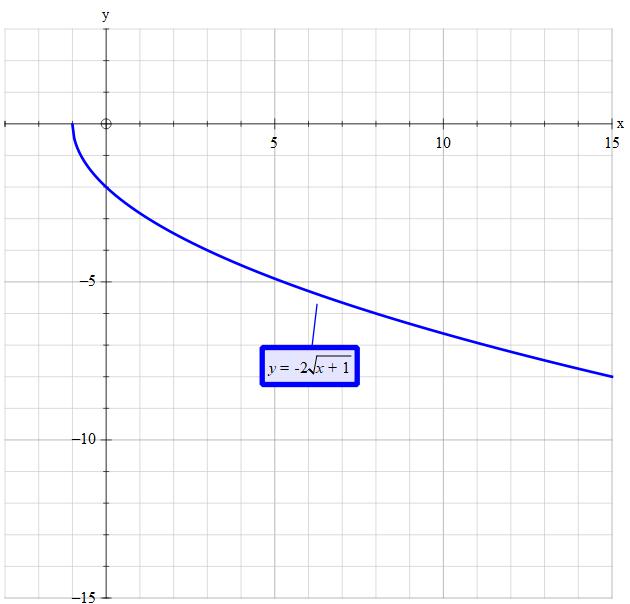
For the solution not to enter the domain of complex numbers the content of the root must never be negative.
Thus the cut off is #x>=-1 #
Deriving the 'cut off point'
The #x+1# 'shifts' ( translate ) the #x# left. In that the x-intercept is:
#y=0=-2sqrt(x+1)#
#sqrt(x+1)=0#
#x=-1 #
You can manipulate the given equation in such a way that you end up with a #ul(color(red)("variant on ") +x=(-y)^2=(+y)^2#. This has the form #sub# as it is a quadratice in #y#
However, the right hand side of #y=-2sqrt(x+1)# will allways be negative so #y# will always be negative. Thus you:
#ul("only have the bottom half of the "sub) #
#color(blue)("In summery you have:")#
The parent graph of #y=x^2# is rotated clockwise #pi/2# and then translated left by 1 on the x-axis. The multiplication by 2 makes it more narrow. The negative means you only have the lower part of the form #sub# ie #y<=0#