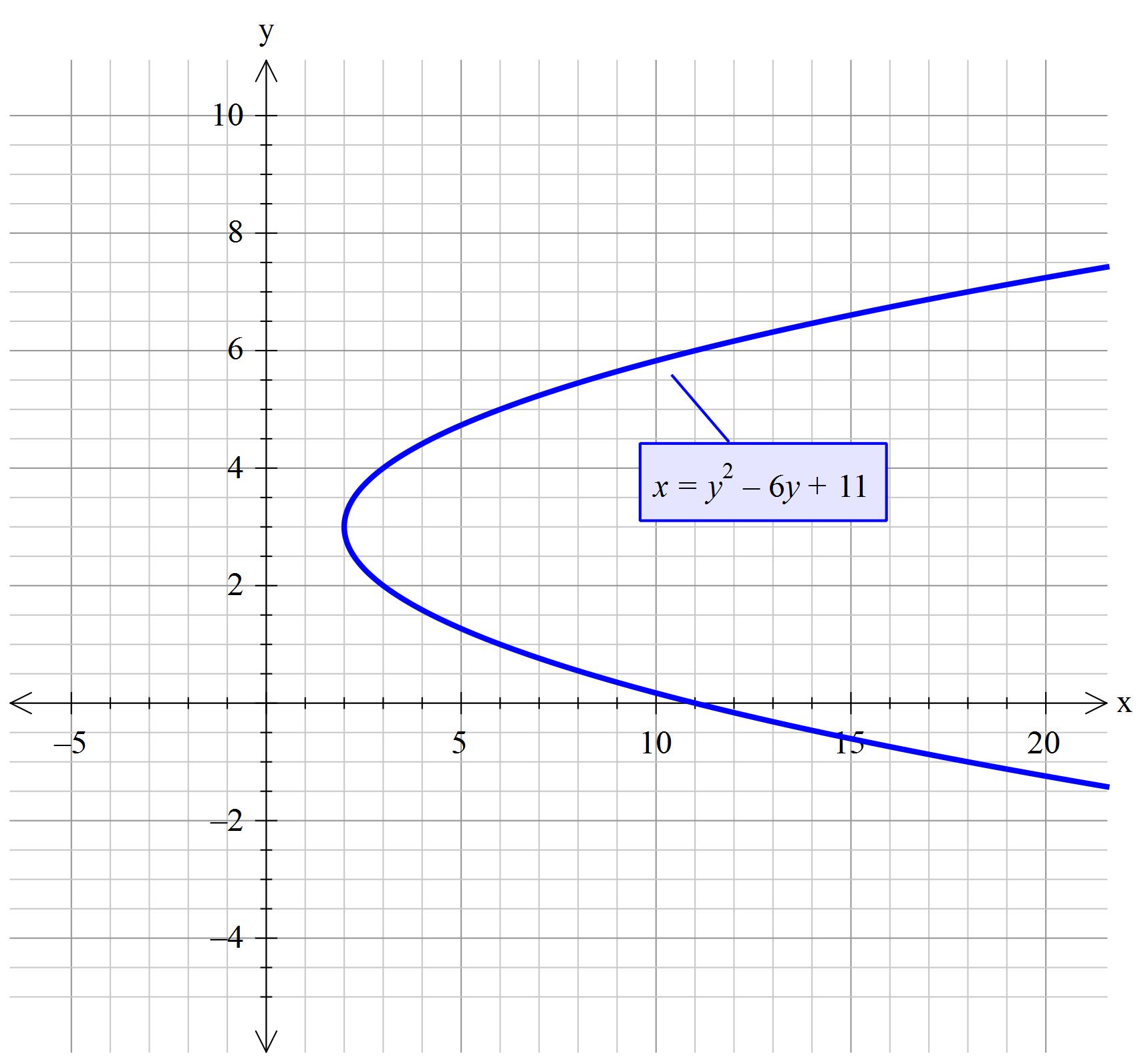
How do you graph #y=sqrt(x-2)+3#?
1 Answer
Jul 28, 2016
See the explanation
Explanation:
Suppose there was an unknown value z
Then
So
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
So in reality we have
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
Lets consider the relationship a different way. The above is such that
Write as
Squaring both sides
If you plotted this then you would have the same graph as