Question

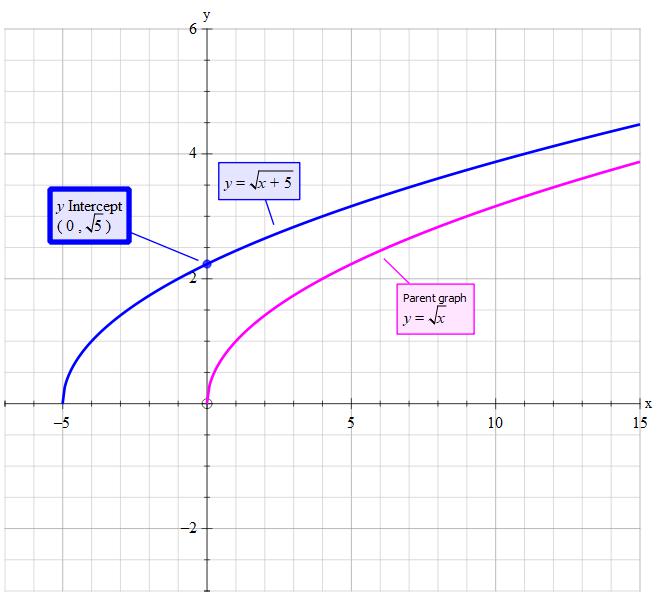
How do you graph `y=sqrt(x+5)`, compare it to the parent graph and what is the domain and range?

Answer 1

Domain `x in[-5,+oo)`
Range `y in [o,+oo)`

Explanation:

Assumption: The parent graph is `y=sqrt(x)`

Although 'true' square root is `+-` it is not stated as such in the question. We only have + so this is what is called the principle square root. The condition `+-` produces the shape `sub` so as we only have the positive half of the condition `+-` we end up with only the top half of the shape `sub`

For the value to NOT go into the complex number set of values the 'content' of the root must not become negative.

Thus the minimum value is such that `x+5=0 =>x=-5`.

The 'action' of changing `y=sqrt(x)" to "y=sqrt(x+5)` 'moves' the graph of `y=sqrt(x)` to the left on the x-axis by 5.

The x-intercept is at `y=0 =sqrt(x+5)` thus `x_("intercept")=-5`
The y-intercept is at `x=0 => y=sqrt(5) ~~2.24` to 2dp
Domain `x in[-5,+oo)`
Range `y in [o,+oo)`