How do you rotate the axes to transform the equation #2x^2-xy-y^2=1# into a new equation with no xy term and then find the angle of rotation?

Question

1930 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-01-09T22:13:12

Use this reference Rotation of Axes

From the reference Rotation of Axes

The given coefficients are #A = 2, B = -1, C = -1, D = E = 0, and F = -1#

According to the reference, to "un-rotate" the given equation, the hyperbola must be rotated by:

#theta = 1/2tan^-1(B/(C - A))#

#theta = 1/2tan^-1(-1/(-1 - 2))#

#theta = 1/2tan^-1(1/3)#

#theta ~~ 9.2^@# or #0.16# radians

Use (9.4.4a) #A' = (A + C)/2 + [(A - C)/2] cos 2θ - B/2 sin 2θ#

#A' = (2 + -1)/2 + [(2 - -1)/2] cos 2θ - -1/2 sin 2θ#

#A' ~~ 2.08#

We know that B will be zero but please feel free to check it.

Use (9.4.4c) #C' = (A + C)/2 + [(C - A)/2] cos 2θ + B/2 sin 2θ#

#C' = (2 + -1)/2 + [(-1 - 2)/2] cos 2θ + -1/2 sin 2θ#

#C' ~~ -1.08#

The new equation is #2.08x^2 - 1.08y^2 = 1#

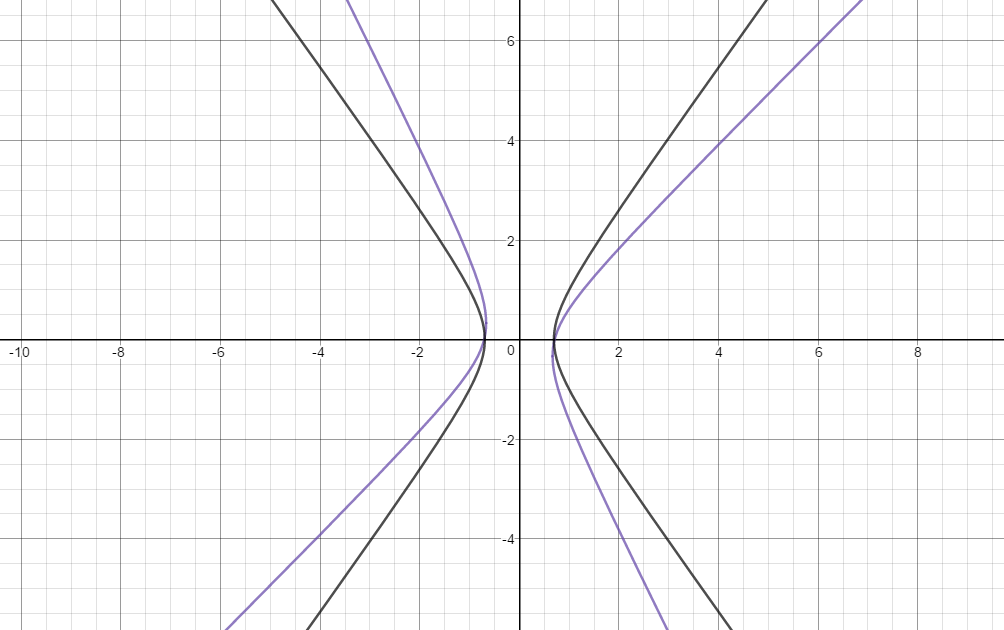
Here is a graph of both equations:

enter image source here