The perimeter of a trapezoid is #42 cm#; the oblique side is #10cm# and the difference between bases is #6 cm#. Calculate: a) The area b) Volume obtained by rotating the trapezoid around the base major?
1 Answer
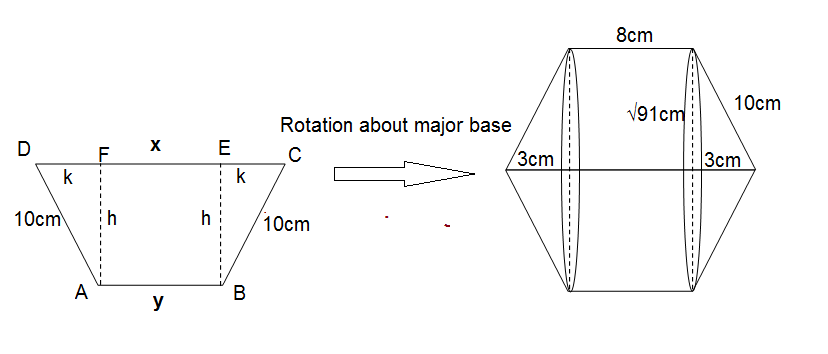
Let us consider an isosceles trapezoid
Its major base
Given
and perimeter
Adding [1] and [2] we get
So
Now
Hence height
So area of the trapezoid
It is obvious that on rotating about major base a solid consisting of two similar cones in two sides and a cylinder at the middle will be formed as shown in above figure.
So total volume of the solid

