Question

Two parallel chords of a circle with lengths of 8 and 10 serve as bases of a trapezoid inscribed in the circle. If the length of a radius of the circle is 12, what is the largest possible area of such a described inscribed trapezoid?

Answer 1

`72*sqrt(2)+9*sqrt(119)~=200.002`

Explanation:

Consider Figs. 1 and 2

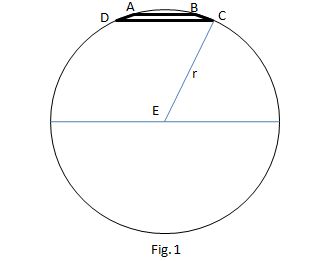
Schematically, we could insert a parallelogram ABCD in a circle, and on condition that sides AB and CD are chords of the circles, in the way of either figure 1 or figure 2.

The condition that the sides AB and CD must be chords of the circle implies that the inscribed trapezoid must be an isosceles one because

• the trapezoid's diagonals (`AC` and `CD`) are equal because
• `A hat B D=B hat A C=B hatD C= A hat C D`
  and the line perpendicular to `AB` and `CD` passing through the center E bisects these chords (this means that `AF=BF` and `CG=DG` and the triangles formed by the intersection of the diagonals with bases in `AB` and `CD` are isosceles).

But since the area of the trapezoid is
`S=(b_1+b_2)/2*h`, where `b_1` stands for base-1, `b_2` for base-2 and `h` for height, and `b_1` is parallel to `b_2`

And since the factor `(b_1+b_2)/2` is equal in the hypotheses of the Figures 1 and 2, what matters is in which hypothesis the trapezoid has a longer height (`h`). In the present case, with chords smaller than the circle's radius, there's no doubt that in the hypothesis of the figure 2 the trapezoid has a longer height and therefore it has a higher area.

According to Figure 2, with `AB=8`, `CD=10` and `r=12`
`triangle_(BEF) -> cos alpha =((AB)/2)/r=(8/2)/12=4/3=1/3`
`->sin alpha = sqrt(1-1/9)=sqrt(8)/3=2sqrt(2)/3`
`->tan alpha=(sin alpha)/cos alpha=(2sqrt(2)/cancel(3))/(1/cancel(3))=2sqrt(2)`
`tan alpha = x/((AB)/2)` => `x=8/cancel(2)*cancel(2)sqrt(2)` => `x=8sqrt(2)`

`triangle_(ECG) -> cos beta=((CD)/2)/r=(10/2)/12=5/12`
`-> sin beta =sqrt (1-25/144)=sqrt(119)/12`
`-> tan beta=(sin beta)/cos beta=(sqrt(119))/cancel(12))/(5/cancel(12))=sqrt(119)/5`
`tan beta=y/((CD)/2)` => `y=10/2*sqrt(119)/5` => `y=sqrt(119)`

Then
`h=x+y`
`h=8sqrt(2)+sqrt(119)`

`S=(b_1+b_2)/2*h=(8+10)/2(8sqrt(2)+sqrt(119))=72sqrt(2)+9sqrt(119)~=200.002`