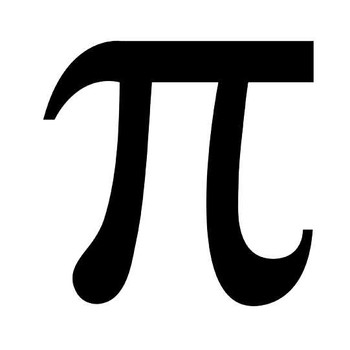
What is the angle between two lines whose direction ratios satisfy following equations?
Find the angle between two lines whose direction ratios satisfy 2l−m+2n=0 and mn+nl+lm=0
Find the angle between two lines whose direction ratios satisfy
1 Answer
The two lines are perpendicular to each other.
Explanation:
Let the direction cosines of the two lines be
Note that if
and the angle
From (1), we get
or
i.e either
if
and if
and
=
=
Hence the two lines are perpendicular to each other.