Question

What is the area of a hexagon with the side that are 1.8 m long?

Answer 1

The area of the hexagon is `8.42`.

Explanation:

The way to find the area of a hexagon is to divide it into six triangles, as shown by the diagram below.

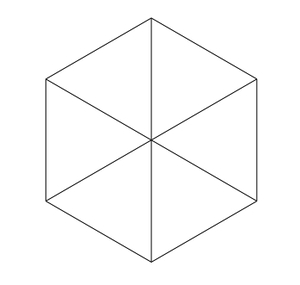
Then, all we need to do is solve for the area of one of the triangles and multiply it by six.

Because it is a regular hexagon, all the triangles are congruent and equilateral. We know this because the central angle is `360˚`, divided into six pieces so that each one is `60˚`. We also know that all of the lines that are inside the hexagon, the ones that make up the side lengths of the triangle, are all the same length. Therefore, we conclude that the triangles are equilateral and congruent.

If the triangle is equilateral, each of its side lengths is the same. It is 1.8 meters long. The formula for the triangle's area is shown below.

`A=1/2sh`

`s` is the side length. `h` is height. We know `s`, and we can use trigonometry to find `h`. The below image shows a 30˚-60˚-90˚ triangle and the formulas for finding the side lengths. We know that our triangle is like this one because all equilateral triangles are 30˚-60˚-90˚, which refers to their three angle measures.

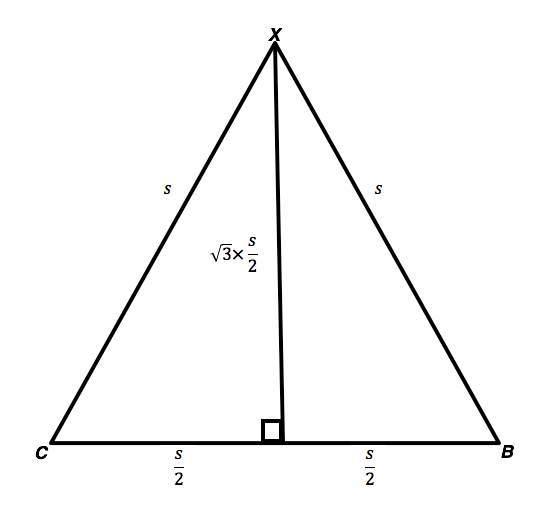
This tells us that the formula for `h` is `sqrt3*s/2`.

`h=sqrt3*1.8/2`
`h~~1.56`

Now, we use the triangle area formula.

`A=1/2*1.56*1.8`
`A= 1.404`

Remember that the hexagon is made of six triangles. Its area is `6` times the triangle's area.

`6*1.404~~8.42`

The area of the hexagon is `8.42`.

If you are interested in a shortcut, you can use the following formula. The longer method above is just useful for understanding the idea behind the formula and how to derive it.

`A=(3sqrt3)/2 * s^2`